Home

Try the Free Math Solver or Scroll down to Tutorials!

Enter expression, e.g. (x^2-y^2)/(x-y)

Enter expression, e.g. x^2+5x+6

Enter expression, e.g. (x+1)^3

Enter a set of expressions, e.g. ab^2,a^2b

Enter equation to solve, e.g. 2x+3=4

Enter equation to graph, e.g. y=3x^2-1

Depdendent Variable

Number of equations to solve:

Equ. #1:

Equ. #2:

Equ. #3:

Equ. #4:

Equ. #5:

Equ. #6:

Equ. #7:

Equ. #8:

Equ. #9:

Solve for:

Enter inequality to solve, e.g. 2x+3>4

Enter inequality to graph, e.g. y<3x^2-1

Dependent Variable

Number of inequalities to solve:

Ineq. #1:

Ineq. #2:

Ineq. #3:

Ineq. #4:

Ineq. #5:

Ineq. #6:

Ineq. #7:

Ineq. #8:

Ineq. #9:

Solve for:

Math solver on your site

Please use this form if you would like
to have this math solver on your website,
free of charge.

Name:

Email:

Your Website:

Msg:

Departmental Syllabus for Finite Mathematics

Instructor Contact Information: This information is section specific and will be given
here in the copy given to each student on the first day of classes.

Course Description: For students not planning to major in the physical sciences, engineering, mathematics, or
computer science. Simple interest, compound interest, and annuities, linear systems and matrices, game theory,
and optimization. Prereq: satisfactory placement test score, or pass M119 or M130. Prerequisite requirements
are strictly enforced. Students not meeting these requirements will be dropped from the class. No student who
has received credit for M141 or M152 with a grade of C or better may subsequently receive credit for M125.
Students who receive a grade of C or better in Math 125 may not subsequently receive credit for M119. (QR)
3 credit hours.

Text: Finite Mathematics, by Waner and Costenoble, Custom 4^th Edition, Brooks Cole Publishers.

Required Companion Website: Enhanced WebAssign: Use the “passkey” that came with your new textbook
and the instructions provided by your instructor.

Calculator: A graphing calculator is required for this course. The Math Department highly recommends and
provides support for the TI-83+ and TI-84+ models. The PIVOT program is required and can be downloaded to
your calculator by the Math Tutorial Center staff. While other calculators may be used with your instructor’s
permission, instructors and tutorial center staff may not be able to provide help on how to use them. Use of cell
phone calculators and calculators with advanced alpha-numeric capabilities, such as the TI-89, is forbidden in
this course.

Grades: Grades will be determined using the grading scale below. Your letter grade is a measure of your
mastery of course material and your fulfillment of course objectives. You should keep all of your graded work
until final grades are posted. The quizzes and other category may contain other material to be specified by the
individual instructor on their individual syllabus.

Grading Scale:

4 Tests for total of	60%
WebAssign Homework	5%
Quizzes and Other	15%
Cumulative Final Exam	20%
Total Possible	100%

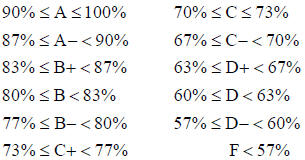
Final Exam: The comprehensive final exam date and time will be stated on the individual instructor’s
syllabus. You need to plan ahead for the date and time of your final exam especially regarding travel
arrangements. There is not a common final for this course. Every instructor writes the final for their class.

All students are required to take the final exam. Students who miss the final
without securing permission ahead of time will fail the course.

Attendance & Make-up Policy: This will be clearly stated on the individual instructor’s syllabus.

Disability Services: If you need course adaptations or accommodations because of a documented disability or
if you have emergency information to share, please contact the Office of Disability Services at 2227 Dunford
Hall at 974-6087.

Math Tutorial Center: The Math Tutorial Center is in rooms 101 and 109 in Temple Court. It provides free
tutoring. Please make use of this free service.

Important Dates:
Add/drop without W deadline	August 28, 2009
Test 1	September 8, 2009
Test 2	October 1, 2009
Drop with W deadline	October 20, 2009
Test 3	October 29, 2009
Drop with WP/WF deadline	November 10, 2009
Test 4	November 24, 2009
Final Exam	Dependent on class meeting time, stated clearly on individual syllabus

Classroom Etiquette: Please be considerate of the instructor and those around you. Come to class on time
and stay the entire period. Turn off cell phones and beepers during class. Do not talk to classmates at
inappropriate times. Refrain from reading newspapers or working on other coursework during class.

Academic Standards of Conduct:
All students are expected to abide by the University Honor Statement. In mathematics classes, violations of
the honor statement include copying another person's work on any graded assignment or test, collaborating on a
graded assignment without the instructor's approval, using unauthorized "cheat sheets" or technical devices
such as calculators, cell phones or computers for graded tests or assignments, or other infractions listed in
"Hilltopics". These violations are serious offenses, subject to disciplinary action that may include failure in a
course and/or dismissal from the University. The instructor has full authority to suspend a student from his/her
class, to assign an "F" in an exercise or examination, or to assign an "F" in the course. See "Hilltopics" for
more complete information. A report of all offenses will be sent to appropriate deans and the Office Student
Judicial Affairs for possible further action.

The Honor Statement

An essential feature of the University of Tennessee is a commitment to maintaining an
atmosphere of intellectual integrity and academic honesty. As a student of the University,
I pledge that I will neither knowingly give nor receive any inappropriate assistance in
academic work, thus affirming my own personal commitment to honor and integrity.

The following schedule is tentative. Each instructor has the option to vary dates and assignments as
they see fit. All skills taught and tested through Test 2 are to be done by hand. Calculators may
be used for dealing with decimals and fractions in certain applications but not on tests. Emphasis
should be on Applications and Communication and Reasoning Exercises. The use of the built-in
financial applications in the calculators is strongly encouraged so focus is on understanding of more
complex financial concepts and their relevance to real life.

Dates	Section	Topic and Suggested Practice Problems
8/20	2.1	Introduction, Syllabus, and Systems of Two Equations in Two Unknowns odd: 1 – 13, 25 – 51 all: 53 – 66
8/25	2.2	Using Matrices to Solve Systems of Equations odd: 1 – 41 all: 51 – 64
8/27	2.3	Applications of Systems of Linear Equations odd: 1 – 13, 17, 19 odd: 25 – 45 all: 47 – 56
9/1	3.1	Matrix Addition and Scalar Multiplication odd: 1 – 27, 37 – 51 all: 53 – 63
9/1	3.2	Matrix Multiplication odd: 1 – 25, 29 – 51, 53 – 57, 63 – 73 all: 77 – 83
9/3	Review
9/8	Test 1	Sections: 2.1, 2.2, 2.3, 3.1, and 3.2
9/10	3.3	Matrix Inversion odd: 1 – 33, 43 – 49, 51 – 57, (Optional fun: 63 – 66) all: 67 – 78
9/15, 17, & 22	3.4	Game Theory all odd
9/22, 24	4.1	Graphing Linear Inequalities odd: 1 – 25, 33 – 43 all: 45 – 58
9/24	4.2	Solving Linear Programming Problems Graphically all odd
9/29	Review
10/1	Test 2	Sections: 3.3, 3.4, 4.1, and 4.2
10/6	4.3	The Simplex Method: Solving Standard Maximization Problems Use PIVOT program for all Simplex. all odd
10/8, 13	4.4	The Simplex Method: Solving General Linear Programming Problems All odd focus on applications
10/20, 22	4.5	The Simplex Method and Game Theory pp: 321 – 325 all: 23 – 28, 39 – 44
10/27	Review
10/29	Test 3	Fixed Date Do Not Push Back Sections 4.3, 4.4, and 4.5
11/3	5.1	Simple Interest all odd, focus on 13 – 44
11/5	5.2	Compound Interest (add continuous compounding) all odd plus continuous focus on 21 – 78
11/10, 12, & 17	5.3	Annuities, Loans, and Bonds Use built-in technology functions in calculators to focus on Applications. Be creative, use multi-phase, real- world examples and problems.
11/19	Review
11/24	Test 4	Sections 5.1, 5.2, and 5.3
12/1	Review	Review for Final Exam
	Final	Date and time by class meeting time per UT Final Exam Schedule