- Home
- INTERMEDIATE ALGEBRA
- Course Syllabus for Algebra I
- Mid-Plains Community College
- FRACTION OF A WHOLE NUMBER
- Systems of Linear Equations
- MATH FIELD DAY
- Course Outline for Finite Mathematics
- Calculus
- Algebra Final Examination
- Math 310 Exam #2
- Review of Trigonometric Functions
- Math 118 Practice test
- Precalculus Review
- Section 12
- Literal Equations
- Calculus Term Definitions
- Math 327A Exercise 2
- Public Key Algorithms II
- Maximizing Triangle Area
- Precalculus I Review for Midterm
- REVIEW OF A FIRST COURSE IN LINEAR ALGEBRA
- Math 6310 Homework 5
- Some Proofs of the Existence of Irrational Numbers
- ALGEBRAIC PROPERTIES OF MATRIX OPERATIONS
- Math 142 - Chapter 2 Lecture Notes
- Math 112 syllabus
- Math 371 Problem Set
- Complex Numbers,Complex Functions and Contour Integrals
- APPLICATIONS OF LINEAR EQUATIONS
- Week 4 Math
- Fractions
- Investigating Liner Equations Using Graphing Calculator
- MATH 23 FINAL EXAM REVIEW
- Algebra 1
- PYTHAGOREAN THEOREM AND DISTANCE FORMULA
- Georgia Performance Standards Framework for Mathematics - Grade 6
- Intermediate Algebra
- Introduction to Fractions
- FACTORINGS OF QUADRATIC FUNCTIONS
- Elementary Algebra Syllabus
- Description of Mathematics
- Integration Review Solutions
- College Algebra - Applications
- A Tip Sheet on GREATEST COMMON FACTOR
- Syllabus for Elementary Algebra
- College Algebra II and Analytic Geometry
- Functions
- BASIC MATHEMATICS
- Quadratic Equations
- Language Arts, Math, Science, Social Studies, Char
- Fractions and Decimals
- ON SOLUTIONS OF LINEAR EQUATIONS
- Math 35 Practice Final
- Solving Equations
- Introduction to Symbolic Computation
- Course Syllabus for Math 935
- Fractions
- Fabulous Fractions
- Archimedean Property and Distribution of Q in R
- Algebra for Calculus
- Math112 Practice Test #2
- College Algebra and Trigonometry
- ALGEBRA 1A TASKS
- Description of Mathematics
- Simplifying Expressions
- Imaginary and Complex Numbers
- Building and Teaching a Math Enhancement
- Math Problems
- Algebra of Matrices Systems of Linear Equations
- Survey of Algebra
- Approximation of irrational numbers
- More about Quadratic Functions
- Long Division
- Algebraic Properties of Matrix Operation
- MATH 101 Intermediate Algebra
- Rational Number Project
- Departmental Syllabus for Finite Mathematics
- WRITTEN HOMEWORK ASSIGNMENT
- Description of Mathematics
- Rationalize Denominators
- Math Proficiency Placement Exam
- linear Equations
- Description of Mathematics & Statistics
- Systems of Linear Equations
- Algebraic Thinking
- Study Sheets - Decimals
- An Overview of Babylonian Mathematics
- Mathematics 115 - College Algebra
- Complex Numbers,Complex Functions and Contour Integrals
- Growing Circles
- Algebra II Course Curriculum
- The Natural Logarithmic Function: Integration
- Rational Expressions
- QUANTITATIVE METHODS
- Basic Facts about Rational Funct
- Statistics
- MAT 1033 FINAL WORKSHOP REVIEW
- Measurements Significant figures
- Pre-Calculus 1
- Compositions and Inverses of Functions
Study Sheets - Decimals
SECTION 3.4 – COMPARING AND CONVERTING DECIMALS AND
FRACTIONS.
Quick facts:
As implied in the previous sections, every fraction can be rewritten
as a decimal. To do
this, just simply divide the numerator of the fraction by the denominator.
Ex. 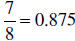 , because
, because
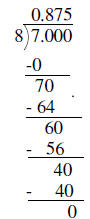
And opposite, a decimal can be written as a
fraction. To do this, the decimal part of the
decimal will be put in the numerator of the fraction, and the denominator will
be derived
from the place value of the last digit of the number being converted.
Ex. 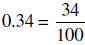 , because the last digit (4) was on the
hundredth place. This fraction can be
, because the last digit (4) was on the
hundredth place. This fraction can be
further simplified. Always try to find the fraction in the simplest form.
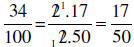
Note: If converting a decimal that contains a whole part,
this whole part would become a
whole part of a mixed number.
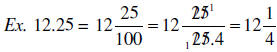
Remember to follow the rules of conversion even for problems like:
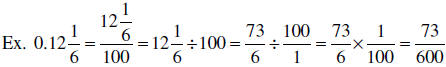
To compare a decimal and a fraction, convert the
fraction into a decimal and than
compare.
Ex. Place a symbol <, > or = between 0.165 and 1/6
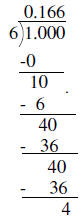
Answer: Because 1/6 is equal to approximately 0.166, which
is greater than 0.165, we can
say: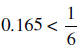
Test yourself:
Convert to a decimal:
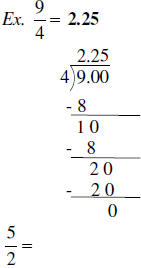 |
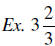 ,round to the
nearest hundredth = 3.67 ,round to the
nearest hundredth = 3.67
|
Convert to a fraction:
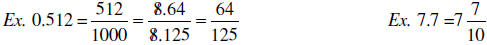
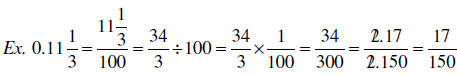
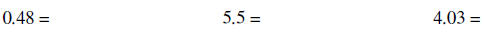
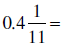
Place a correct symbol between the numbers:
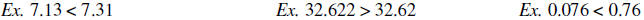
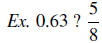
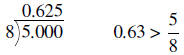

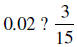
Challenge Yourself:
It might be useful for the future for you to know the basic conversions between
fractions
and decimals, as they are widely used in real life. Think of change, for example
a quarter
(which is $ 1/4), can be also written as $0.25.
Getting accustomed with the conversion table (that you can find in Appendix of
this
chapter) simplifies the process of converting fractions to decimals by
eliminating of the
repetitive dividing.
Assignment:
Textbook Chapter 3.6 (p.162): Exercises 1 – 69 every fourth problem



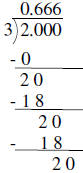
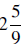 , round to the
nearest thousandth
, round to the
nearest thousandth