Math 327A Exercise 2

1. The decreasing sequence 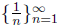, i.e., the decreasing sequence 1,1/2.1/3,1/4,1/5,1/6,...,1/n,...
converges to 0. The sequence {1/3, 1/2, 1, 1/6, 1/5, 1/4, 1/9, 1/8, 1/7, ...} is not decreasing,
but it still converges to 0. By what principle from assignment 1 can you conclude that that
sequence converges (to 0)?

Definition: 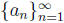is a convergent sequence if it has a limiting value.

Definition: A subsequence 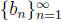of a sequence 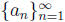is any sequence obtained from
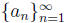by eliminating some of the terms in the a_n-sequence. For example, the sequence of
odd positive integers is a subsequence of the sequence of all positive integers.

2.(a). Suppose that the sequence 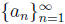is a list of the rational numbers between 0 and 1 (in
some order). For instance, it might be the sequence 1,1/2,1/3,2/3,1/4,3/4,1/5,2/5,3/5,4/5,...,
in which each rational is in lowest terms, and where we list the numbers with smaller de-
nominators first.

Show that every real number between 0 and 1 is the limit of some convergent subsequence
of 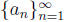. (Let r be a real number, 0 < r < 1. Using problem 5 of assignment 1, we
know that there is a rational number between 0 and r. That number appears once in the
list 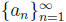; say it is the number 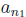. Let 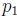be the point half way between 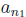and r. We
know that there are an infinite number of rational numbers between 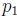and r, i.e., an infinite
number of terms of the sequence 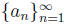between 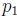and r. Let 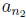be the first term of the
sequence after 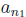that lies between 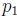and r. Then 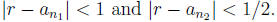Next
find a term 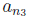after that is more than half way from to r. Then .
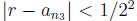Continuing in this way, you should be able to find a subsequence 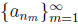of the sequence
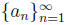such that 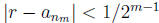. That subsequence converges to r.)

(b). Find a subsequence of the rational number sequence 0, 1, 1/2, 1/3, 2/3, 1/4, 3/4, 1/5, 2/5, 3/5, 4/5, ...
that converges to 2/3. (The sequence 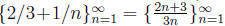converges to 2/3. Take the
subsequence where you drop the terms whose indices n are divisible by 3. Confirm that that
subsequence is also a subsequence of the rational number sequence given at the beginning of
the problem.)

Definition: Let S be a set of real numbers. S has an upper bound if there is an integer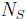
which is greater than (or equal to) each number in S, i.e., 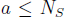holds for each number
.

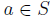The ordered set of real numbers has the property that each set of real numbers with an
upper bound has a least upper bound, and each set of real numbers with lower bound has a
greatest upper bound.

3. Let 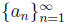be an increasing sequence of real numbers that has an upper bound, i.e., the
set of numbers occurring in the sequence has an upper bound, and let m be the least upper
bound of the set of numbers in the sequence. Show that the sequence converges to m.

It is a fact that 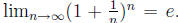. Using that fact, show the facts given in exercise 4
below.

4(a). Compute the equality

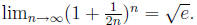
(b). Compute

(c). Compute

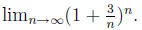
Showing that a sequence 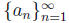has limit m is the same as showing that the sequence
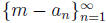has limit 0. (See exercise 1 of assignment 1.)

5. Suppose that 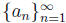converges to m and that 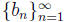converges to k. Show that
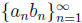converges to mk. (Hint: Show that 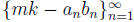converges to 0. To do that,
write mk − 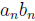as 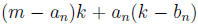and show that the summands (m − 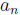)k and
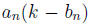determine sequences 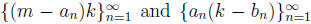that converge to 0.
(In dealing with the second sequence, you may assume the fact (or prove the fact) that if a
sequence 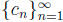consists of a set of numbers that has an upper bound and a lower bound
(that property holds when the sequence converges, for instance) and if a sequence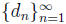
converges to 0, then the sequence 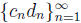converges to zero.)

6. Show that 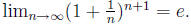(Use problem 5).