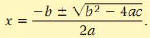- Home
- INTERMEDIATE ALGEBRA
- Course Syllabus for Algebra I
- Mid-Plains Community College
- FRACTION OF A WHOLE NUMBER
- Systems of Linear Equations
- MATH FIELD DAY
- Course Outline for Finite Mathematics
- Calculus
- Algebra Final Examination
- Math 310 Exam #2
- Review of Trigonometric Functions
- Math 118 Practice test
- Precalculus Review
- Section 12
- Literal Equations
- Calculus Term Definitions
- Math 327A Exercise 2
- Public Key Algorithms II
- Maximizing Triangle Area
- Precalculus I Review for Midterm
- REVIEW OF A FIRST COURSE IN LINEAR ALGEBRA
- Math 6310 Homework 5
- Some Proofs of the Existence of Irrational Numbers
- ALGEBRAIC PROPERTIES OF MATRIX OPERATIONS
- Math 142 - Chapter 2 Lecture Notes
- Math 112 syllabus
- Math 371 Problem Set
- Complex Numbers,Complex Functions and Contour Integrals
- APPLICATIONS OF LINEAR EQUATIONS
- Week 4 Math
- Fractions
- Investigating Liner Equations Using Graphing Calculator
- MATH 23 FINAL EXAM REVIEW
- Algebra 1
- PYTHAGOREAN THEOREM AND DISTANCE FORMULA
- Georgia Performance Standards Framework for Mathematics - Grade 6
- Intermediate Algebra
- Introduction to Fractions
- FACTORINGS OF QUADRATIC FUNCTIONS
- Elementary Algebra Syllabus
- Description of Mathematics
- Integration Review Solutions
- College Algebra - Applications
- A Tip Sheet on GREATEST COMMON FACTOR
- Syllabus for Elementary Algebra
- College Algebra II and Analytic Geometry
- Functions
- BASIC MATHEMATICS
- Quadratic Equations
- Language Arts, Math, Science, Social Studies, Char
- Fractions and Decimals
- ON SOLUTIONS OF LINEAR EQUATIONS
- Math 35 Practice Final
- Solving Equations
- Introduction to Symbolic Computation
- Course Syllabus for Math 935
- Fractions
- Fabulous Fractions
- Archimedean Property and Distribution of Q in R
- Algebra for Calculus
- Math112 Practice Test #2
- College Algebra and Trigonometry
- ALGEBRA 1A TASKS
- Description of Mathematics
- Simplifying Expressions
- Imaginary and Complex Numbers
- Building and Teaching a Math Enhancement
- Math Problems
- Algebra of Matrices Systems of Linear Equations
- Survey of Algebra
- Approximation of irrational numbers
- More about Quadratic Functions
- Long Division
- Algebraic Properties of Matrix Operation
- MATH 101 Intermediate Algebra
- Rational Number Project
- Departmental Syllabus for Finite Mathematics
- WRITTEN HOMEWORK ASSIGNMENT
- Description of Mathematics
- Rationalize Denominators
- Math Proficiency Placement Exam
- linear Equations
- Description of Mathematics & Statistics
- Systems of Linear Equations
- Algebraic Thinking
- Study Sheets - Decimals
- An Overview of Babylonian Mathematics
- Mathematics 115 - College Algebra
- Complex Numbers,Complex Functions and Contour Integrals
- Growing Circles
- Algebra II Course Curriculum
- The Natural Logarithmic Function: Integration
- Rational Expressions
- QUANTITATIVE METHODS
- Basic Facts about Rational Funct
- Statistics
- MAT 1033 FINAL WORKSHOP REVIEW
- Measurements Significant figures
- Pre-Calculus 1
- Compositions and Inverses of Functions
Imaginary and Complex Numbers
Section 10.8 Imaginary and Complex Numbers
| The number i i is the
unique number for which |
Ex. Find…

Powers of i
Ex. Find…



Section 11.1 Solving Quadratic Equations
What is a quadratic equation?
The standard form of a quadratic equation is ax2 + bx + c = 0 , where a ≠ 0, b
and
c are real numbers
Examples:
| The Principle of Zero Products
An equation ab=0 is true if and only a=0 or b= 0 , or
both .(A |
Ex. Solve x2− x = 12
Ex. Solve 12x2 − 5x − 2 = 0
| The Principle of Square Roots
For any real number k, if x2 =k, then
|
Ex. Solve



| The Principle of Square Roots (Generalized
Form ) For any real number k and any
algebraic expression X. |
Ex. Solve…


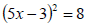
Ex. Given f (x) = x2 − 6x + 2
a. Find f (−3)
b. Where is f (x) = −3
Ex. Given the function f (x) = x2 − 6x + 2
a. Find the y-intercept
b. Find the x-intercepts
Completing the Square
Ex. Solve x2 −10x + 25 = 100
| How to complete the square: 1. Start with x2 + bx 2. Evaluate 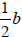 3. Evaluate 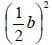 4. Combine 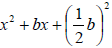 5. Factor, end up with 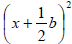 |
Ex. Complete the square


How to solve the equation
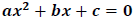 by first completing the square by first completing the square1. If necessary, move c to the right-hand side of the equation 2. If necessary, divide both sides of the equation by a 3. Complete the square on the left-hand side 4. Balance the equation by adding 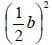 to
right-hand side. to
right-hand side.5. Factor and solve using Principal of Square Roots |
Ex. Solve by completing the square


Section 11.2 Quadratic Formula
Developing the formula…
| The Quadratic Formula
The solutions of
|
Ex. Solve…
2x2 −9x + 5 = 0
Ex. Solve…
4x2 = 3+ 7x
3x2 + 2x = 7
12x2 − 5x − 2 = 0
Ex. Given 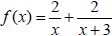 , where is f
(x) = 1?
, where is f
(x) = 1?
Ex. Given 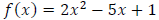
a. Find the y-intercept
b. Find the x-intercept(s), if they exist.
Which method to use?
Factoring
Principal of Square Roots
Completing the Square
Quadratic Formula
Section 11.3 Formulas and Applications
Ex. Solve for d: 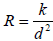
Ex. Solve for t:Y = rt 2 − st
Ex. Sandi’s Subaru travels 280 mi averaging a certain speed. If the car had gone
5
mph faster, the trip would have taken 1 hr less. Find Sandi’s average speed.
| Distance | Rate | Time |



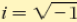 and i2
=-1
and i2
=-1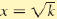 or
or
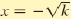 .
.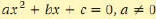 are given by
are given by