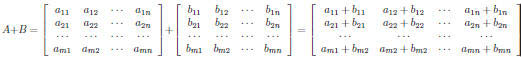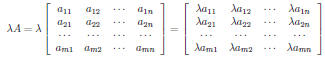- Home
- INTERMEDIATE ALGEBRA
- Course Syllabus for Algebra I
- Mid-Plains Community College
- FRACTION OF A WHOLE NUMBER
- Systems of Linear Equations
- MATH FIELD DAY
- Course Outline for Finite Mathematics
- Calculus
- Algebra Final Examination
- Math 310 Exam #2
- Review of Trigonometric Functions
- Math 118 Practice test
- Precalculus Review
- Section 12
- Literal Equations
- Calculus Term Definitions
- Math 327A Exercise 2
- Public Key Algorithms II
- Maximizing Triangle Area
- Precalculus I Review for Midterm
- REVIEW OF A FIRST COURSE IN LINEAR ALGEBRA
- Math 6310 Homework 5
- Some Proofs of the Existence of Irrational Numbers
- ALGEBRAIC PROPERTIES OF MATRIX OPERATIONS
- Math 142 - Chapter 2 Lecture Notes
- Math 112 syllabus
- Math 371 Problem Set
- Complex Numbers,Complex Functions and Contour Integrals
- APPLICATIONS OF LINEAR EQUATIONS
- Week 4 Math
- Fractions
- Investigating Liner Equations Using Graphing Calculator
- MATH 23 FINAL EXAM REVIEW
- Algebra 1
- PYTHAGOREAN THEOREM AND DISTANCE FORMULA
- Georgia Performance Standards Framework for Mathematics - Grade 6
- Intermediate Algebra
- Introduction to Fractions
- FACTORINGS OF QUADRATIC FUNCTIONS
- Elementary Algebra Syllabus
- Description of Mathematics
- Integration Review Solutions
- College Algebra - Applications
- A Tip Sheet on GREATEST COMMON FACTOR
- Syllabus for Elementary Algebra
- College Algebra II and Analytic Geometry
- Functions
- BASIC MATHEMATICS
- Quadratic Equations
- Language Arts, Math, Science, Social Studies, Char
- Fractions and Decimals
- ON SOLUTIONS OF LINEAR EQUATIONS
- Math 35 Practice Final
- Solving Equations
- Introduction to Symbolic Computation
- Course Syllabus for Math 935
- Fractions
- Fabulous Fractions
- Archimedean Property and Distribution of Q in R
- Algebra for Calculus
- Math112 Practice Test #2
- College Algebra and Trigonometry
- ALGEBRA 1A TASKS
- Description of Mathematics
- Simplifying Expressions
- Imaginary and Complex Numbers
- Building and Teaching a Math Enhancement
- Math Problems
- Algebra of Matrices Systems of Linear Equations
- Survey of Algebra
- Approximation of irrational numbers
- More about Quadratic Functions
- Long Division
- Algebraic Properties of Matrix Operation
- MATH 101 Intermediate Algebra
- Rational Number Project
- Departmental Syllabus for Finite Mathematics
- WRITTEN HOMEWORK ASSIGNMENT
- Description of Mathematics
- Rationalize Denominators
- Math Proficiency Placement Exam
- linear Equations
- Description of Mathematics & Statistics
- Systems of Linear Equations
- Algebraic Thinking
- Study Sheets - Decimals
- An Overview of Babylonian Mathematics
- Mathematics 115 - College Algebra
- Complex Numbers,Complex Functions and Contour Integrals
- Growing Circles
- Algebra II Course Curriculum
- The Natural Logarithmic Function: Integration
- Rational Expressions
- QUANTITATIVE METHODS
- Basic Facts about Rational Funct
- Statistics
- MAT 1033 FINAL WORKSHOP REVIEW
- Measurements Significant figures
- Pre-Calculus 1
- Compositions and Inverses of Functions
ON SOLUTIONS OF LINEAR EQUATIONS
| Homework Section 1.3: 4,14,34,48,50,26*,46* | ||||||
| MATRIX. A rectangular array of numbers is called
a matrix
A matrix with m rows and n columns
is called a m × n matrix. A matrix with one column is a column |
||||||
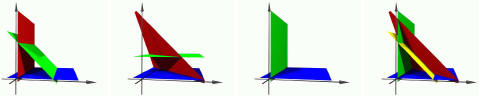
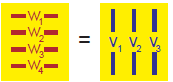
ROW AND COLUMN PICTURE. Two interpretations
Row picture: each bi is the dot
product of a row vector |
||||||
| EXAMPLE. The system of linear equations
is equivalent to
The augmented matrix (separators for clarity)
In this case, the row vectors of A are
The column vectors are
Row picture:
Column picture:
|
||||||
|
||||||
|
||||||
| MURPHYS LAW. ”If anything can go wrong, it will go wrong”. ”If you are feeling good, don’t worry, you will get over it!” ”For Gauss-Jordan elimination, the error happens early in the process and get unnoticed.
|
||||||
| MURPHYS LAW IS TRUE. Two equations could
contradict each other. Geometrically, the two planes do not intersect. This is possible if they are parallel. Even without two planes being parallel, it is possible that there is no intersection between all three of them. It is also possible that not enough equations are at hand or that there are many solutions. Furthermore, there can be too many equations and the planes do not intersect.
|
||||||
| RELEVANCE OF EXCEPTIONAL CASES. There are
important applications, where ”unusual” situations happen: For example in medical tomography, systems of equations appear which are ”ill posed”. In this case one has to be careful with the method. The
linear equations are then obtained from a method called the Radon
|
||||||
| MATRIX ALGEBRA. Matrices can be added, subtracted
if they have the same size:
They can also be scaled by a scalar λ:
|
||||||





 with
with
 .
.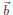 is a sum of
scaled column vectors
is a sum of
scaled column vectors 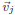 .
.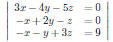
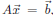 where A is a
coefficient
where A is a
coefficient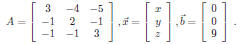
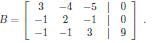
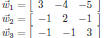
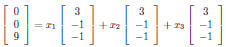
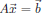 with m equa-
with m equa-