- Home
- INTERMEDIATE ALGEBRA
- Course Syllabus for Algebra I
- Mid-Plains Community College
- FRACTION OF A WHOLE NUMBER
- Systems of Linear Equations
- MATH FIELD DAY
- Course Outline for Finite Mathematics
- Calculus
- Algebra Final Examination
- Math 310 Exam #2
- Review of Trigonometric Functions
- Math 118 Practice test
- Precalculus Review
- Section 12
- Literal Equations
- Calculus Term Definitions
- Math 327A Exercise 2
- Public Key Algorithms II
- Maximizing Triangle Area
- Precalculus I Review for Midterm
- REVIEW OF A FIRST COURSE IN LINEAR ALGEBRA
- Math 6310 Homework 5
- Some Proofs of the Existence of Irrational Numbers
- ALGEBRAIC PROPERTIES OF MATRIX OPERATIONS
- Math 142 - Chapter 2 Lecture Notes
- Math 112 syllabus
- Math 371 Problem Set
- Complex Numbers,Complex Functions and Contour Integrals
- APPLICATIONS OF LINEAR EQUATIONS
- Week 4 Math
- Fractions
- Investigating Liner Equations Using Graphing Calculator
- MATH 23 FINAL EXAM REVIEW
- Algebra 1
- PYTHAGOREAN THEOREM AND DISTANCE FORMULA
- Georgia Performance Standards Framework for Mathematics - Grade 6
- Intermediate Algebra
- Introduction to Fractions
- FACTORINGS OF QUADRATIC FUNCTIONS
- Elementary Algebra Syllabus
- Description of Mathematics
- Integration Review Solutions
- College Algebra - Applications
- A Tip Sheet on GREATEST COMMON FACTOR
- Syllabus for Elementary Algebra
- College Algebra II and Analytic Geometry
- Functions
- BASIC MATHEMATICS
- Quadratic Equations
- Language Arts, Math, Science, Social Studies, Char
- Fractions and Decimals
- ON SOLUTIONS OF LINEAR EQUATIONS
- Math 35 Practice Final
- Solving Equations
- Introduction to Symbolic Computation
- Course Syllabus for Math 935
- Fractions
- Fabulous Fractions
- Archimedean Property and Distribution of Q in R
- Algebra for Calculus
- Math112 Practice Test #2
- College Algebra and Trigonometry
- ALGEBRA 1A TASKS
- Description of Mathematics
- Simplifying Expressions
- Imaginary and Complex Numbers
- Building and Teaching a Math Enhancement
- Math Problems
- Algebra of Matrices Systems of Linear Equations
- Survey of Algebra
- Approximation of irrational numbers
- More about Quadratic Functions
- Long Division
- Algebraic Properties of Matrix Operation
- MATH 101 Intermediate Algebra
- Rational Number Project
- Departmental Syllabus for Finite Mathematics
- WRITTEN HOMEWORK ASSIGNMENT
- Description of Mathematics
- Rationalize Denominators
- Math Proficiency Placement Exam
- linear Equations
- Description of Mathematics & Statistics
- Systems of Linear Equations
- Algebraic Thinking
- Study Sheets - Decimals
- An Overview of Babylonian Mathematics
- Mathematics 115 - College Algebra
- Complex Numbers,Complex Functions and Contour Integrals
- Growing Circles
- Algebra II Course Curriculum
- The Natural Logarithmic Function: Integration
- Rational Expressions
- QUANTITATIVE METHODS
- Basic Facts about Rational Funct
- Statistics
- MAT 1033 FINAL WORKSHOP REVIEW
- Measurements Significant figures
- Pre-Calculus 1
- Compositions and Inverses of Functions
Precalculus I Review for Midterm
1. Let a(x) = 2x - 5 and b(x) = -x + 1. Solve a(x) = b(x), a(x) b(x). What are
the
graphs of a(x), b(x)? Where do the graphs of a(x) and b(x) intersect?
Solution: x = 2 and x≤2. Graphs of a(x), b(x) are straight lines which intersect
at
the point (2,-1).
2. For the function f(x) = (x + 1)2 - 4 find out if the graph opens up or down,
what
are to coordinates of the vertex, what are the x- and y-intercepts if any.
Solution: Writing out the formula we get f(x) = x2 + 2x - 3 which shows that
the
parabola opens up. The coordinates of the vertex are given for free - (-1,-4).
The
x-intercepts are x = 1 and x = -3. The y-intercept is (0,-3).
3. For the function g(x) = 3x2 + 4x - 1 find out if the graph opens up or down,
what
are to coordinates of the vertex, what are the x- and y-intersects if any.
Solution: The graph opens up. The coordinates of the vertex are (-2/3,-7/3).
The
x-intercepts are 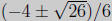 . The y-intercept is (0,-1).
. The y-intercept is (0,-1).
4. Find a quadratic function that has the x- intercepts x = -2 and x = 1 and
whose
graph passes through the point (0,-2).
Solution: f(x) = (x + 2)(x - 1) = x2 + x - 2.
5. Find a quadratic function whose graph has the vertex at (2, 4) and has an
x-intercept
at x = 0.
Solution: f(x) = -(x - 2)2 + 4.
6. A landscape engineer has 200 feet of border to enclose a rectangular pond.
What
dimensions will result in the largest pond?
Solution: The dimensions are 50x50.
7. A special window in the shape of a rectangle with semicircles at each end is
to be
constructed so that the outside dimensions are 100 feet in length. Find the
dimensions
of the rectangle that maximizes its area.
Solution: The dimensions of the rectangle are
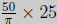 .
.
For the following polynomials:
a) find the x- and y-intercepts of each polynomial
b) determine whether the graph of f touches or crosses the x-axis at each x-
intercept
c) find the power function that the graph of f resembles for large values of |x|
d) determine the behaviour of f near each of the x-intercepts
e) list all zeros with their multiplicities
8. f(x) = x(x + 2)(x + 4)
Solution: The x-intercepts are x = 0, x = -2 and x = -4. The y-intercept is
(0, 0).
The graph crosses the x-axis at each intercept since the multiplicities are 1.
The power
function we're looking for is f(x) = x3. At x = 0 we have f(x) 8x, at x = -2 we
8x, at x = -2 we
have f(x)  -4(x + 2) and at x = -4 we have f(x)
-4(x + 2) and at x = -4 we have f(x)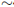 8(x + 4). The zeros are x = 0
8(x + 4). The zeros are x = 0
with multiplicity 1, x = -2 with multiplicity 1 and x = -4 with multiplicity
1.
9. f(x) = (x - 2)(x + 2)2
Solution: The x-intercepts are x = 2, x = -2. The y-intercept is (0,-8). The
graph
crosses the x-axis at x = 2 since the multiplicity is 1 and touches the x-axis
at x = -2
since the multiplicity is 2. The power function we're looking for is f(x) = x3.
At x = 2
we have f(x)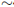 16(x - 2), at x = -2 we have f(x) -4(x + 2)2. The zeros are x =
2
16(x - 2), at x = -2 we have f(x) -4(x + 2)2. The zeros are x =
2
with multiplicity 1, x = -2 with multiplicity 2.
10. f(x) = (x - 1)2(x + 3)(x + 1)
Solution: The x-intercepts are x = 1, x = -3 and x = -1. The y-intercept is
(0, 3).
The graph crosses the x-axis at x = -3 and x = -1 since the multiplicities are
1
and touches the x-axis at x = 1 since the multiplicity is 2. The power function
we're
looking for is f(x) = x4. At x = 1 we have f(x)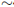 8(x - 1)2, at x = -3 we have
8(x - 1)2, at x = -3 we have
f(x) 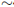 -32(x + 3) and at x = -1 we have f(x)
-32(x + 3) and at x = -1 we have f(x)
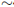 8(x + 1). The zeros are x = 1 with
8(x + 1). The zeros are x = 1 with
multiplicity 2, x = -3 with multiplicity 1 and x = -1 with multiplicity 1.
11. f(x) = -2x3 + 4x2
Solution: The x-intercepts are x = 0, x = 2. The y-intercept is (0, 0). The
graph
crosses the x-axis at x = 2 since the multiplicity is 1 and touches the x-axis
at x = 0
since the multiplicity is 2. The power function we're looking for is f(x) =
-2x3. At
x = 0 we have f(x) 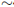 4x2, at x = 2 we have f(x)
4x2, at x = 2 we have f(x)
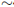 -8(x - 2). The zeros are x = 0
-8(x - 2). The zeros are x = 0
with multiplicity 2, x = 2 with multiplicity 1.
12. f(x) = (x - 2)2(x + 4)2
Solution: The x-intercepts are x = 2 and x = -4. The y-intercept is (0, 64).
The
graph touches the x-axis at each x-intercept since the multiplicities are 2. The
power
function we're looking for is f(x) = x4. At x = 2 we have f(x)
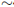 36(x-2)2, at x =
-4
36(x-2)2, at x =
-4
we have f(x) 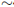 36(x + 4)2. The zeros are x = -4 with multiplicity 2, x = 2 with
36(x + 4)2. The zeros are x = -4 with multiplicity 2, x = 2 with
multiplicity 2.
For the following functions nd the domain, horizontal, vertical or oblique
asymp-
totes.
13. 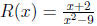
Solution: Domain is 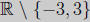 . Vertical asymptotes are x = -3 and x = 3. The
. Vertical asymptotes are x = -3 and x = 3. The
horizontal asymptote is y = 0.
14. 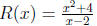
Solution: Domain is 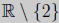 . Vertical asymptotes is x = 2. The oblique asymptote
is
. Vertical asymptotes is x = 2. The oblique asymptote
is
y = x + 2.
15. 
Solution: Domain is 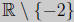 . x = -2 is a vertical asymptote. y = 1 is a
horizontal
. x = -2 is a vertical asymptote. y = 1 is a
horizontal
asymptote.
16. 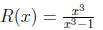
Solution: Domain is 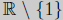 . x = 1 is a vertical asymptote. y = 1 is a
horizontal
. x = 1 is a vertical asymptote. y = 1 is a
horizontal
asymptote.
Solve the following inequalities
17. 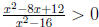
Solution: The solution is 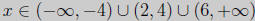 .
.
18. 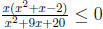
Solution: The solution is 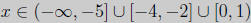 .
.


