Math Proficiency Placement Exam

The following is a list of competencies addressed on the exam.

The student will be able to:

1. Simplify real number expressions using order of operations.
2. Graph points and intervals of points on the real number line.
3. Classify real numbers as rational, irrational, integer, and/or non-integer values.
4. Identify real number properties such as commutativity, associativity, distributive law, identities, and inverses.
5. Translate verbal expressions into algebraic expressions.
6. Simpify algebraic expressions by combining like terms.
7. Evaluate algebraic expressions.
8. Solve linear equations.
9. Construct and use a linear model to solve an application problem.
10. Plot points in the Cartesian plane.
11. Graph a straight line.
12. Determine the x and y-intercepts of a line.
13. Determine the slope of a line.
14. Determine if lines are parallel or perpendicular using slope.
15. Use the point-slope formula to find the equation of a line.
16. Use the slope-intercept form of a line to determine its slope and y-intercept.
17. Determine whether an expression represents a function using various techniques, including the Vertical Line Test.
18. Evaluate functions.
19. Find the sum, difference, product, and quotient of functions.
20. Form the composition of functions.
21. Construct and use models to relate quantities that vary directly, inversely, and/or jointly.
22. Simplify exponential expressions using rules for exponents.
23. Express numbers in scientific notation.
24. Identify the terms and degree of a polynomial.
25. Add, subtract, and multiply polynomials.
26. Factor algebraic expressions using such techniques as common factoring, factoring by grouping, difference of squares, difference of cubes, sum of cubes, and trial and error techniques for trinomials.
27. Solve quadratic equations using factoring techniques.
28. Construct and use a quadratic model to solve an application problem.
29. Simplify, multiply, divide, add, and subtract rational algebraic expressions.
30. Simplify radical expressions using rules for radicals.
31. Determine the domain and range of an exponential function and sketch its graph.
32. Use an exponential model to solve an application problem.
33. Determine the inverse of a function, if appropriate.
34. Determine the domain and range of a logarithmic function and sketch its graph.
35. Use a logarithmic model to solve an application problem.
36. Use properties of logarithms to expand and evaluate expressions.
37. Solve exponential and logarithmic equations algebraically.
38. Solve systems of equations involving two equations and two variables.

An excellent source to review before attempting the exam is Schaum’s Outline of
Intermediate Algebra by Ray Steege and Kerry Bailey (1997, McGraw-Hill).

The format of the exam is multiple-choice. Here are some sample questions. These
sample questions are designed to give you an idea of the format of the exam. In no way
is this an exhaustive list of topics covered on the exam.

1. The expression ( y − 2)^2 is equivalent to

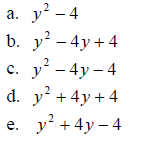
The correct answer is B.

2. Solve the given equation for the variable.

6 + 2m = 18
a. -6
b. 12
c. 24
d. 6
e. 4

The correct answer is D.

3. Factor the expression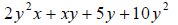

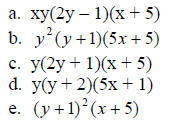
The correct answer is C.

4. Solve the system of equations.

3x – 5y = 7
-x + y = -1
a. (-1, -2)
b. (-3, 2)
c. (1, -4)
d. (2, 1)
e. (-1, -4)

The correct answer is A.

5. Simplify the expression

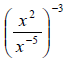
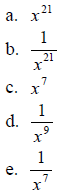
The correct answer is B.