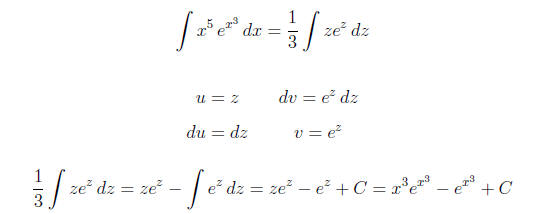- Home
- INTERMEDIATE ALGEBRA
- Course Syllabus for Algebra I
- Mid-Plains Community College
- FRACTION OF A WHOLE NUMBER
- Systems of Linear Equations
- MATH FIELD DAY
- Course Outline for Finite Mathematics
- Calculus
- Algebra Final Examination
- Math 310 Exam #2
- Review of Trigonometric Functions
- Math 118 Practice test
- Precalculus Review
- Section 12
- Literal Equations
- Calculus Term Definitions
- Math 327A Exercise 2
- Public Key Algorithms II
- Maximizing Triangle Area
- Precalculus I Review for Midterm
- REVIEW OF A FIRST COURSE IN LINEAR ALGEBRA
- Math 6310 Homework 5
- Some Proofs of the Existence of Irrational Numbers
- ALGEBRAIC PROPERTIES OF MATRIX OPERATIONS
- Math 142 - Chapter 2 Lecture Notes
- Math 112 syllabus
- Math 371 Problem Set
- Complex Numbers,Complex Functions and Contour Integrals
- APPLICATIONS OF LINEAR EQUATIONS
- Week 4 Math
- Fractions
- Investigating Liner Equations Using Graphing Calculator
- MATH 23 FINAL EXAM REVIEW
- Algebra 1
- PYTHAGOREAN THEOREM AND DISTANCE FORMULA
- Georgia Performance Standards Framework for Mathematics - Grade 6
- Intermediate Algebra
- Introduction to Fractions
- FACTORINGS OF QUADRATIC FUNCTIONS
- Elementary Algebra Syllabus
- Description of Mathematics
- Integration Review Solutions
- College Algebra - Applications
- A Tip Sheet on GREATEST COMMON FACTOR
- Syllabus for Elementary Algebra
- College Algebra II and Analytic Geometry
- Functions
- BASIC MATHEMATICS
- Quadratic Equations
- Language Arts, Math, Science, Social Studies, Char
- Fractions and Decimals
- ON SOLUTIONS OF LINEAR EQUATIONS
- Math 35 Practice Final
- Solving Equations
- Introduction to Symbolic Computation
- Course Syllabus for Math 935
- Fractions
- Fabulous Fractions
- Archimedean Property and Distribution of Q in R
- Algebra for Calculus
- Math112 Practice Test #2
- College Algebra and Trigonometry
- ALGEBRA 1A TASKS
- Description of Mathematics
- Simplifying Expressions
- Imaginary and Complex Numbers
- Building and Teaching a Math Enhancement
- Math Problems
- Algebra of Matrices Systems of Linear Equations
- Survey of Algebra
- Approximation of irrational numbers
- More about Quadratic Functions
- Long Division
- Algebraic Properties of Matrix Operation
- MATH 101 Intermediate Algebra
- Rational Number Project
- Departmental Syllabus for Finite Mathematics
- WRITTEN HOMEWORK ASSIGNMENT
- Description of Mathematics
- Rationalize Denominators
- Math Proficiency Placement Exam
- linear Equations
- Description of Mathematics & Statistics
- Systems of Linear Equations
- Algebraic Thinking
- Study Sheets - Decimals
- An Overview of Babylonian Mathematics
- Mathematics 115 - College Algebra
- Complex Numbers,Complex Functions and Contour Integrals
- Growing Circles
- Algebra II Course Curriculum
- The Natural Logarithmic Function: Integration
- Rational Expressions
- QUANTITATIVE METHODS
- Basic Facts about Rational Funct
- Statistics
- MAT 1033 FINAL WORKSHOP REVIEW
- Measurements Significant figures
- Pre-Calculus 1
- Compositions and Inverses of Functions
Integration Review Solutions
Divide x by x + 7 using long division. The quotient is 1 and the remainder -7.
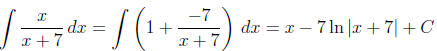
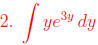
Use integration by parts:
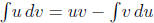 .
.
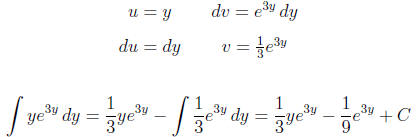
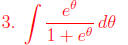
Use a u-substitution: 
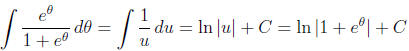
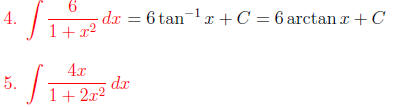
Use a u-substitution: 

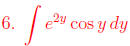
Use integration by parts:
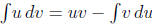 .
.
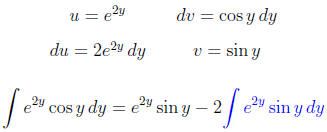
Now use integration by parts again for
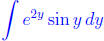
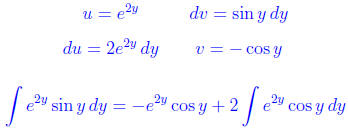
Put everything together:
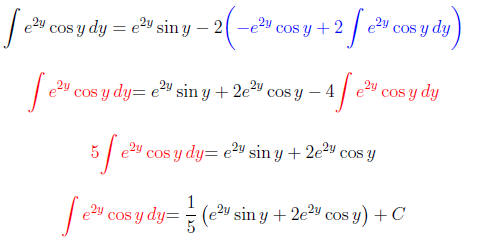
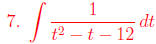
Use partial fractions.
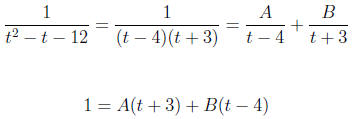
Plug in t = -3 to see that
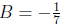 . Plug in t = 4 to see that
. Plug in t = 4 to see that
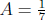 .
.
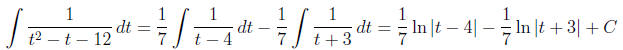
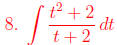
Divide t2 + 2 by t + 2 using long division. The quotient is t - 2 and the remainder is 6.
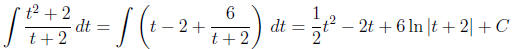
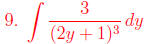
Use a u-substitution. u = 2y + 1
 du = 2 dy
du = 2 dy
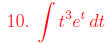
Use integration by parts multiple times, or use the D/I
chart. In the first integration
by parts iteration, u = t3 and dv = et dt. Therefore, put t3 in the D column and
put et in
the I column. The letter D stands for derivative and I for integral. Fill in the
columns
accordingly until you get to a 0.
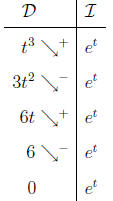
Draw diagonal arrows and label with alternating positive
and negative signs. Multiply
along the diagonals, and add or subtract the resulting terms depending on the
sign of the
arrow. Don't forget to add C.
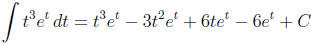
Use integration by parts:
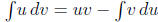 .
.
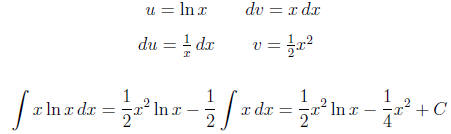
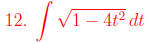
Use a trig. substitution:
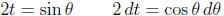
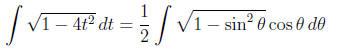
If we are careful, we see that this integral equals
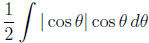 . Let's assume for now
. Let's assume for now
that cosθ > 0. Is this assumption valid? Think about the range of θ in the
substitution.
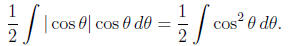
Use trig. identities to simplify.
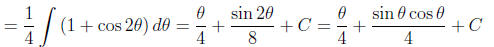
From our substitution 2t = sinθ , we deduce:
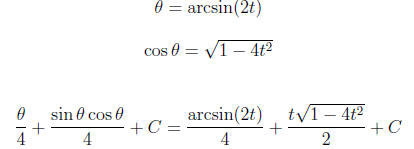
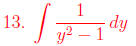
Use partial fractions.
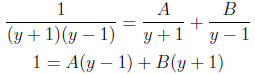
Substitute y = 1 to see that
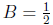 and y = -1 to see that
and y = -1 to see that
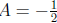 .
.
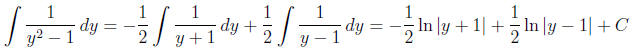
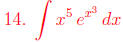
Do a z-substitution, then do integration by parts.
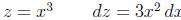 .
.